Derivation

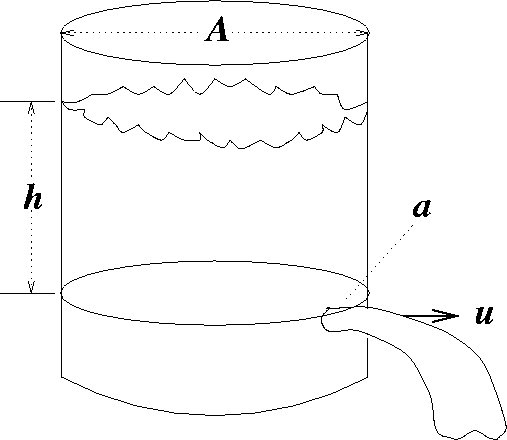
The Torricelli model for the draining bucket is based on two physical concepts:

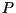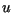1. The Bernoulli relationship between pressure, $P$, density, $\rho$ and speed, $u$ for fluid along a streamline:
   
   $$\Delta P = \frac12 \rho u^2,$$
   
   

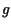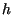2. The hydrostatic relationship between changes in pressure over the height of a fluid column and gravity (acceleration $g$), fluid density $\rho$ and the height $h$ of the column:
   
   $$\Delta P = \rho g h.$$
   
   

Equating these two gives a relationship between height of the fluid above the hole and the speed at which it spews forth,

$$u = \sqrt{2 g h}.$$

The because the volume lost of fluid in the bucket must equal the flux of fluid through the bucket's hole (with area 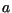$a$),

$$\frac{dV}{dt} = - u a,$$

and using the fact that (for a bucket with regular sides and constant cross section in height) 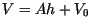$V=A h + V_0$,

$$- u a = \frac{dV}{dt} = \frac{d}{dt} \left[A h + V_0 \right] = A\frac{dh}{dt} .$$

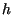Substituting in the relationship $u=\sqrt{2gh}$ from above gives a differential equation for height of the fluid, $h$, as a function of time:

$$\frac{dh}{dt} = -\frac{a\sqrt{2 g}}{A} \sqrt{h} . \hspace{1.5in} (*)$$